Measuring Resistivity
When to use eddy current instruments to measure resistivity
Resistivity can be measured using a number of different methods to include reflectance spectroscopy, transmittance spectroscopy, and ellipsometry. In these spectroscopic methods, the relationship between the optical properties and the resistivity of the material depends on the nature of the material and the experimental setup. Therefore, a calibration or reference measurement may be required to establish the relationship.
Each of these technologies has its advantages and disadvantages. Eddy current technology is usually most appropriate when:
- Non-contact technology is needed
- High precision and accuracy is needed
- The material’s properties make other methods of evaluation ineffective
- Material thickness is known or can easily be acquired. (Note: nearly all non-contact thickness methods require reference information or special calibration.)
Resistivity and sheet resistance
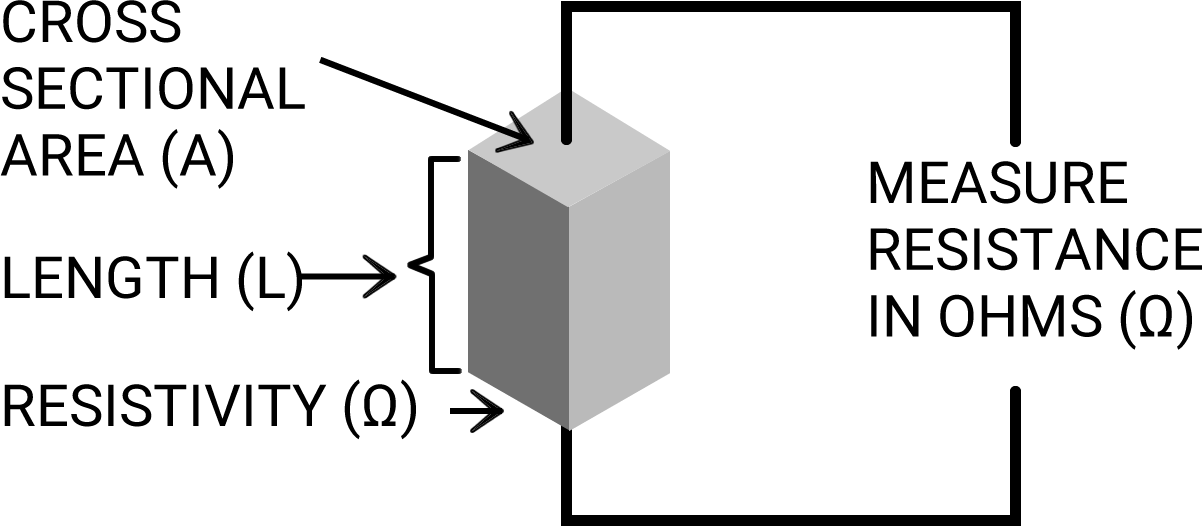
The electrical resistance (R) of an object is measured in Ohms and represented by the Greek letter Ω (omega). The resistance of a metal or a semiconductor can be measured using an ohmmeter (a common function on benchtop multimeters). The measurement of an object’s resistance can be taken with such a meter independent of the dimensions of the object or the object’s internal composition.
Figure 1: Resistance of an object

Three factors contribute to an object’s overall resistance. It is intuitive that a longer object is more resistive to electrical flow, as the electrons have farther to travel. Similarly, a narrower object is more resistive to flow, as there are fewer parallel pathways for the electrons to travel down. Finally, an object that has a material more intrinsically resistant to the flow of electrons will be more resistive. This intrinsic characteristic of a material that makes it conductive to the flow of electrons is called resistivity.
Therefore, we can say that electrical resistance (R) of an object is a result of three factors:
- Cross-sectional area of an object (A)
- Length of the object (L)
- Intrinsic properties of the material the object is composed of—resistivity (ρ)
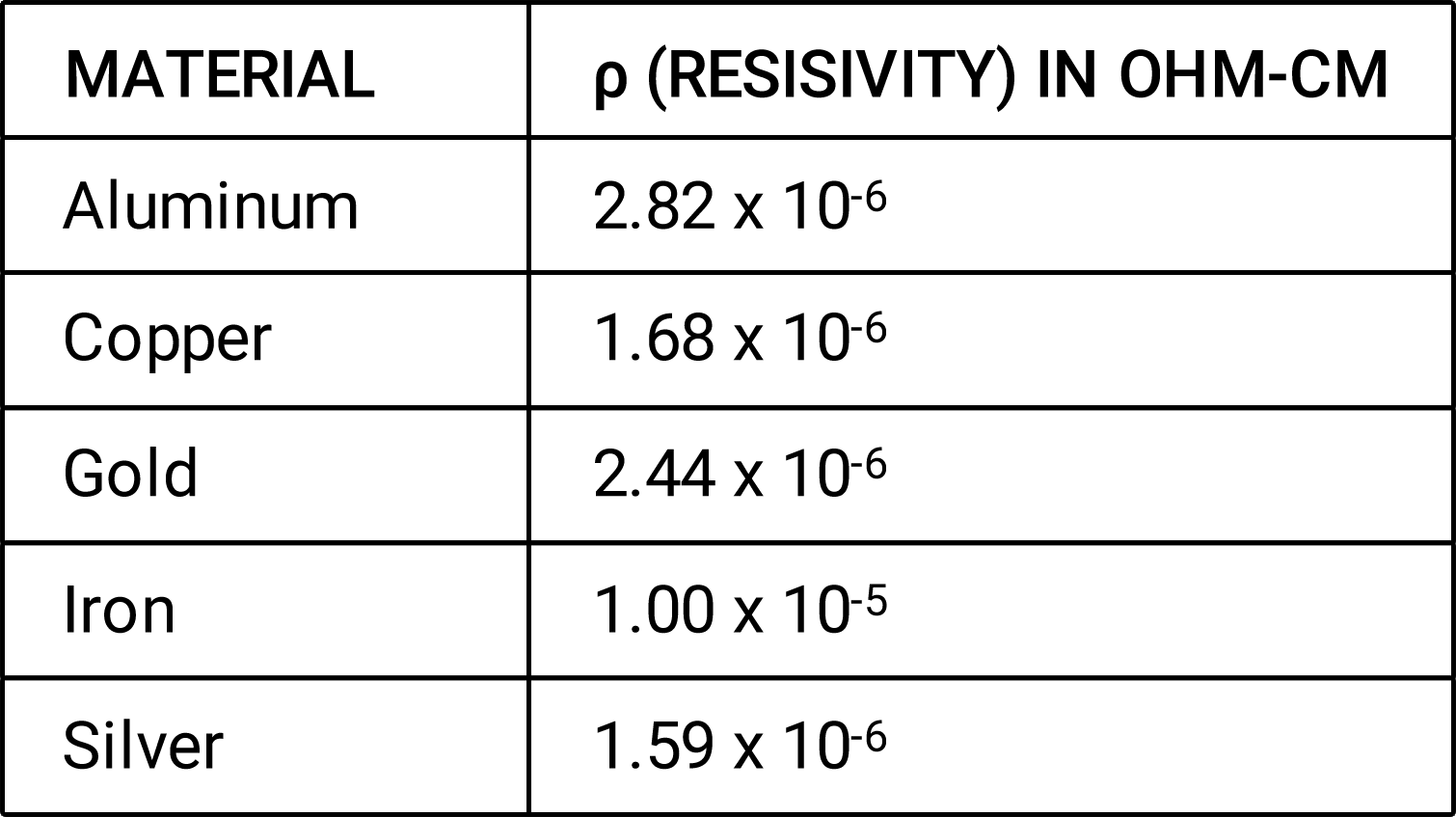
To determine the resistivity of various materials, objects composed of different materials but with the same dimensions were tested for their resistance. Table 1 shows the resistivities of some common materials.
Table 1: Resistivities of various materials
From these observations about the three factors of resistance, we conclude that resistance equals resistivity (ρ) times length (L), divided by area (A), as demonstrated by Figure 2 and Equation 1.
Figure 2: Resistance is a function of resistivity (ρ), length (L), and area (A)
Equation 1: Formula for resistance
R = ρxLA.
In order to find the sheet resistance of an object, think of a square shape cut out of a doped silicon wafer, conductive film sheet, or a coated glass panel. We don’t want to have to worry about length and width along the entire plane of the material. This can be avoided by assuming a square shape. Now let’s describe the square as having a length (L), width (W), and thickness (T), as shown in Figure 3 below.
Figure 3: Sheet of resistive material
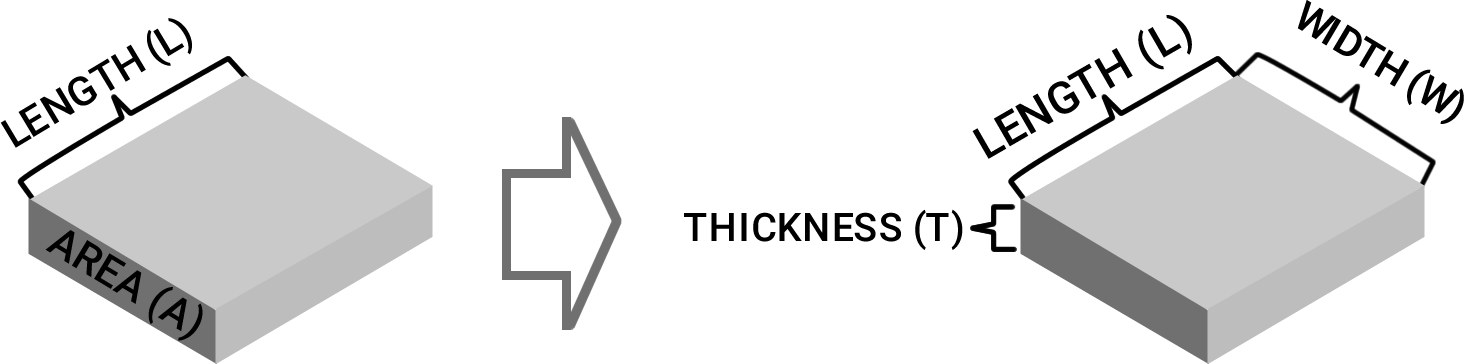
Next, let’s take our formula that relates length, area, and resistivity to resistance and transform it. This is done by substituting area (A) with width (W) times thickness (T).
Equation 2: Transforming resistance equation
R = ρxL A ⇒ R = ρxL LxW
Finally, since the material in this example is a perfect square, we can substitute length (L) for width (W). This results in the equation: Sheet resistance (Rs) equals resistivity (ρ) divided by thickness (T).
Equation 3: Formula for sheet resistance
Let L =W and consider Rs
Rs = ρxL TxL ⇒ Rs = ρ T
Knowing that sheet resistance equals resistivity divided by thickness, we can conclude that resistivity equals sheet resistance times thickness. Therefore, to determine resistivity, the thickness must be known and the resistivity calculated by multiplying the sheet resistance by the thickness.
Equation 4: Formula for resistivity
Rs = ρ T ⇒ ρ = Rs x T
Delcom software allows the user to input the thickness into the software. The software then multiplies all subsequent sheet resistance readings by that thickness and displays a mathematically computed resistivity.
Example: Consider a material of thickness T = 0.02 cm and sheet resistance of Rs = 3.0 ohms/square. This material would have a volume resistivity of .06 ohms-cm, which is 0.02 cm multiplied by 3.0 ohms/square.
Units of measurement
Delcom uses the metric unit of measurement for resistivity: ohm-cm.
Applications that measure/monitor resistivity
- Transparent Conductors
- Displays
- Wires, Meshes, & Grids
- Carbon Nanotubes
- Graphene
- Thin Film Solar
- LEDs
- Low E Glass
- Smart Glass
- Thin Film Heaters
- Semiconductor Wafers
- Crystalline Solar
- Packaging
- Microwave Susceptors
- Film Capacitors
- Printed Electronics
- Batteries & Storage
- EMI & EMS
- Auto Mirrors
- Transparent Conductors
- Displays
- Wires, Meshes, & Grids
- Carbon Nanotubes
- Graphene
- Thin Film Solar
- LEDs
- Low E Glass
- Smart Glass
- Thin Film Heaters
- Semiconductor Wafers
- Crystalline Solar
- Packaging
- Microwave Susceptors
- Film Capacitors
- Printed Electronics
- Batteries & Storage
- EMI & EMS
- Auto Mirrors
How eddy current instruments measure resistivity
Eddy current meters work by introducing a current into the sensor’s inductor coil(s). This generates an oscillating magnetic field. When a conductive material is introduced into the magnetic field, a circular flow of electrons begins to move in the conductive layer due to magnetic induction. Eddy current technology earned its name because this flow of electrons is reminiscent of eddy current swirls in a body of water.
An eddy current instrument works by coupling the material to be tested to an amplitude stabilized oscillator. The power absorbed by the material in the oscillating magnetic field is proportional to the conductivity of the material. This power level is delivered as voltage to an analog-to-digital converter (ADC). This voltage is scaled from 0.0001 to 2.0000 voltage direct current (VDC). For a standard range meter, .0001 VDC is equal to .0001 Siemens/square.
To get to resistivity, the eddy current meter digitally reciprocates sheet conductance to display the material’s sheet resistance (measured in ohms/square). At this point, the user input material thickness (measured in microns) is multiplied by the material’s sheet resistance. The resulted calculated value is then displayed for the user in ohms-cm or micro-ohms-cm.
Configuring your instrument to display resistivity
In order to display resistivity to the user, the Delcom meter must be told what the thickness of the material is. The meter then performs the appropriate calculations and displays the resistivity of the material. This requires the user to know the thickness of the material and input this thickness value into the meter.
There are multiple methods available to determine the thickness of a material. These are listed here in order from most to least practical:
- Ask the supplier of your conductive material what the thickness of the material is
- If your material is silicon wafer, a foil, or some other material that often has standard sizes, assume the material is uniformly of the stated standard thickness
- Use a precision thickness meter/method such as a surface profilometers, ellipsometers, spectroscopic reflectometers, interferometers, weight measuring, Quartz Crystal Microbalances(QCMs), and non-contact eddy current instruments.
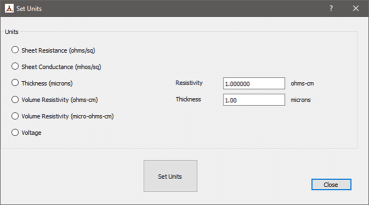
In order to measure resistivity with a Delcom meter, the user must have access to Delcom software. The software might only need to be used once, but it is necessary to set the units of measurement to be displayed:
- Open the Delcom software.
- Go to the main menu bar and choose Edit and then Units.
- Select the radio button titled Volume Resistivity.
Figure 4: Setting units of measurement in the Delcom software
Upon completion of these steps, all subsequent readings by the meter on any of the user interfaces will be displayed in resistivity. This includes the LCD display, regardless of whether the PC is connected to the interface module or not. The user can revert to other units of measurement at any time by editing units under the settings menu.

 Veteran Owned
Veteran Owned Veteran Owned
Veteran Owned


